Achromatization by Combined Volume Bragg Gratings & Surface Diffraction Gratings
Over the last decades phase masks have found numerous applications including imaging, encryption, beam shaping, and mode conversion. There are two typical methods of making such masks. The first one controls the local geometrical path length by generating a contoured surface while the second method changes the local refractive index in the bulk of a photosensitive medium such as lithium niobate or photosensitive glass. Both methods can be employed to generate phase masks with almost any profile. However, because the phase shift is induced by changing the local optical path length these phase masks are inherently limited to use at a specific wavelength, which limits the range of potential applications. To extend this range achromatic phase masks have been previously produced utilizing complex fabrication approaches.
Leonid Glebov and his group recently developed a new holographic element that can be utilized as achromatic phase plate. It is generated by encoding stepped or grey level phase mask profiles into transmitting volume Bragg grating (TBG) and is named “holographic phase mask” (HPM). It was shown that HPMs can produce identical to a standard phase plate diffracted phase profiles over a wide range of wavelengths as long as the Bragg condition of the volume grating is satisfied.
The HPM also utilizes the diffraction characteristics of a TBG, which can diffract up to 100% of a beam into a single order, and can diffract over a broad range of wavelengths by changing the angle of incidence (with the diffraction efficiency depending on the wavelength and strength of the grating). The high angular selectivity of a TBG also allows for several TBGs to be multiplexed into the same element with little to no cross-talk between gratings; each grating is accessed by altering the beam’s angle of incidence onto the element.

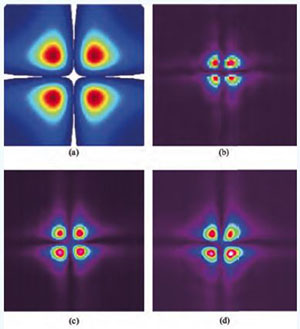
It is important to note that a Bragg grating is the simplest volume hologram, which, unlike more complex holograms, can diffract narrow wavelength band incoming at a given angle, without distorting the beam profile. By encoding phase levels which cover a macroscopic area, the HPM acts locally as a standard TBG with a given phase shift. Thus the HPM will diffract in the same manner as a standard TBG except at the relatively small number of phase discontinuities, and the diffracted beam’s phase profile will match the encoded phase level profile regardless of incident wavelength. The HPM therefore acts as a spectrally addressable phase mask. To simplify fabrication and to provide a clear demonstration of the phenomenon binary phase profiles were used to create HPM, but the approach is fully applicable for multi-level phase masks as well. To show the unique properties, a four-sector binary phase mask was chosen as an example. The expected far field intensity profile for a beam passing through the center of a four-sector regular binary phase mask consists of a four-lobed clover pattern. As shown in the figure, for three very different Bragg wavelengths (632.8 nm, 975 nm, and 1064 nm), the diffracted beam profiles exhibited the predicted four-lobed pattern. This clearly confirms that the binary phase profile is being preserved in the diffracted order for an extremely broad range of wavelengths.
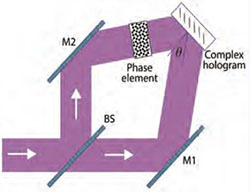
True achromatization of HPMs can be achieved with the concept of pairing the Bragg grating with two surface gratings According to the well-known grating dispersion equation a surface grating with a given period (ΛSG) will diffract normally incident light an angle (θ) in different orders as a function of wavelength (λ). Simultaneously, from coupled wave theory, a VBG will diffract light highly efficient at the Bragg condition. In order for the diffraction angles to match and therefore the surface and volume gratings to work together the condition comes to:
2ΛVBG = ΛSG
Therefore, if the surface grating period is double the period of the volume Bragg grating, then any first order diffraction by normally incident light will be at the corresponding Bragg condition of the volume Bragg grating and that will hold for any wavelength. If an identical surface grating is added in a mirror orientation to the transmitting volume Bragg grating like shown in the figure, the dispersion will be completely canceled and the outgoing beam will be re-collimated. Applying this concept to the holographic phase mask alleviated the need for angle tuning so the Bragg condition can be met, making it a fully achromatic phase element.
The experiments conducted with such system demonstrated highly efficient mode conversion from a Gaussian to a TEM11 mode for a range of more than 300 nm without the need for any angular adjustments. Prof. Glebov’s group continues to implement VBGs in many high- and low-power laser and photonics applications.
Authors

